1.03 Concatenation and the fundamental group
Video
Below the video you will find accompanying notes and some pre-class questions.
- Previous video: 1.02 Paths, loops, and homotopies.
- Next video: 1.04 Examples and simply-connectedness.
- Index of all lectures.
Notes
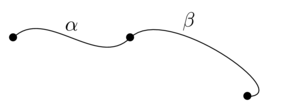
Concatenation
(0.10) Let \(X\) be a topological space and suppose \(\alpha,\beta\colon[0,1]\to X\) are paths such that \(\alpha(1)=\beta(0)\). The concatenation \(\beta\cdot\alpha\) is the path \[(\beta\cdot\alpha)(t)=\begin{cases}\alpha(2t)&\mbox{ if }t\in[0,1/2]\\\beta(2t-1)&\mbox{ if }t\in[1/2,1].\end{cases}\]
The fundamental group
(3.49) Given a topological space \(X\) and a basepoint \(x\in X\), write \(\Omega_xX\) for the set of all loops in \(X\) based at \(x\). The relation \(\simeq\) that two loops \(\alpha,\beta\in\Omega_xX\) are based-homotopic is an equivalence relation. We write \(\pi_1(X,x)\) for the set of equivalence classes \(\Omega_xX/\simeq\) of loops up to based homotopy.
We can make \(\pi_1(X,x)\) into a group under concatenation: if \([\alpha]\in\pi_1(X,x)\) denotes the homotopy class of the loop \(\alpha\) then \[[\beta]\cdot[\alpha]=[\beta\cdot\alpha].\]
The operation \([\beta]\cdot[\alpha]=[\beta\cdot\alpha]\) defines a group structure on \(\pi_1(X,x)\). In particular:
- it is well-defined on homotopy classes,
- it is associative,
- it has an identity (the identity element is the constant loop \(\epsilon(t)=x\)),
- each homotopy class of loops \([\gamma]\) has an inverse \([\bar{\gamma}]\), where \(\bar{\gamma}(t)=\gamma(1-t)\) is the loop which runs around \(\gamma\) in reverse.
(8.30) We will show that the constant loop \(\epsilon\) is an identity for concatenation on \(\pi_1(X,x)\); the other parts are left as exercises.
The concatenation \(\gamma\cdot\epsilon\) stays at \(x\) for time \(1/2\) and then moves around \(\gamma\) at double-speed. This is not equal to the loop \(\gamma\), but it only differs in the way it is parametrised. By playing with the parametrisation, we can construct a homotopy \(\gamma_s\) from this concatenation to the original loop \(\gamma\). \[ \gamma_s(t)=\begin{cases} \epsilon(t)&\mbox{ if }t\leq 1/2(1-s)\\ \gamma((2-s)t+s-1)&\mbox{ if }t\geq 1/2(1-s) \end{cases} \] (10.46) To understand this formula, observe that at the stage \(s\) in the homotopy, the loop \(\gamma_s\) stays at \(x\) for time \(1/2(1-s)\) (\(1/2\) when \(s=0\) and \(0\) when \(s=1\)) then moves around \(\gamma\) at \((2-s)\) times the speed of the original loop (twice as fast when \(s=0\), the same speed as the original when \(s=1\)). Here is a picture of the domain of the homotopy:

(14.46) This homotopy is continuous by one of the exercises we will do in class when we learn about topological spaces; it is a homotopy rel endpoints since \(\gamma_s(0)=\gamma_s(1)=x\) for all \(s\); and it satisfies \(\gamma_0=\gamma\cdot\epsilon\) and \(\gamma_1=\gamma\). A similar homotopy works for \(\epsilon\cdot\gamma\).
Pre-class questions
- Suppose that \(\alpha_t\) is a homotopy between \(\alpha_0\) and \(\alpha_1\) and \(\beta_t\) is a homotopy between \(\beta_0\) and \(\beta_1\). Can you write down a homotopy between \(\beta_0\cdot\alpha_0\) and \(\beta_1\cdot\alpha_1\)? This verifies one of the claims from the lemma in the video/notes: which claim?
Navigation
- Previous video: 1.02 Paths, loops, and homotopies.
- Next video: 1.04 Examples and simply-connectedness.
- Index of all lectures.